The real cost of any decision is what you forego by making that choice. In economics, the cost of a decision based on the cost of the next best option is called the opportunity cost. At its most poetic, Henry David Thoreau put it thus: ‘the price of anything is the amount of life you pay for it’.

At first blush that may seem a little extreme, but it is a truth. We are all finite beings and will one day run out of life. Time really is the ultimate constraint. If we had an infinite amount of time, we could do everything we desired and have time left over to enjoy it all.

Over the last few articles of this series, we have looked at two-tier scheduling, the resource management problem and the direction of a solution, as well as the structure of the VMO (value management office) to bring these innovations in schedule and resource management into being. All these components play a part in delivering more of your goal within the time you have available to do it. However, as necessary as they are to optimising individual projects, by themselves, they are not sufficient to make more intelligent decisions between the different initiatives arising from your strategy and business plan.

In the world of finance, portfolio management is the art and science of making decisions about investment mix and policy, matching investments to objectives, asset allocation for individuals and institutions, and balancing risk against performance. Project portfolio management (PPM) refers to a process used by project management organisations to analyse the potential return on undertaking a project. Managers can use PPM to see the big picture and make decisions about what’s out and what’s in.

‘The price of anything is the amount of
life you pay for it’—Henry David Thoreau

In both the world of finance and the world of projects, the goal is to maximise the return on resources invested in creating value from an idea. Typically, this will be calculated using two related ideas: the net present value (NPV) and the internal rate of return (IRR). The idea behind NPV is to calculate all of the cash flowing in and out of your investment over a predetermined time to tell you what those flows are worth if all of it were converted into a single equivalent capital amount today.

The NPV on any given project is heavily dependent on the assumptions of the positive and negative cash flows, as well as the rate of interest used to discount the value of the money you will only realise in the future. Hence, the technique used to determine NPV is called ‘discounted cash flow’. IRR is related to NPV in that the IRR is a target baseline discount (interest) rate that calculates the net present value (NPV) of all future cash flows from a particular project to come out as zero. Therefore, if you can achieve more than the internal rate of return, the project is—technically, at least—worth taking on.

Working with limiting factors
As described in my recent article on constraint accounting, no less a figure than the late Stanford Professor Charles T Horngren stated that ‘relevant information is the predicted future costs and revenues that will differ among alternative actions. The existence of a limiting factor changes the basic assumptions underlying the cost and revenue opportunity of a particular action.’ Thus, if the particular ‘action’ we are talking about is selecting which projects from a portfolio will deliver the best return on investment, then rank ordering on the basis of NPV is not sufficient.

What we need to do to properly evaluate the best decision, based on Horngren’s idea of a ‘limiting factor’ is to introduce the idea of project octane. What I mean by project octane is how much NPV the project delivers for per unit of the critically constrained resource (CCR). Because projects are always competing for finite resources.

Let’s imagine you are evaluating a portfolio of 10 projects, each of which has been evaluated on the basis of its NPV. You are satisfied with the assumptions your team have made with regards to the value each project will deliver and they have been very diligent with their scheduling. You are able to drill into all resource requirements and what they have produced confirms your intuition that the critically constrained resource is your pool of solution architects. You can readily ramp up all other resource types but, for the technology platform you are using, you are simply unable to contract more than 80 solution architects.

When you first look at the portfolio, it looks like the table below:

Scenario 1:

Based on the availability of only 80 of your critically constrained resource (CCR), we can only, strictly speaking, do the first two projects, and at a squeeze, perhaps get the third one in. Total NPV for the executable portfolio is therefore between $1.9bn and $2.7bn.

Scenario 2:

If however you add to the table the project octane for each project—that is, how much NPV is delivered for each unit of the CCR—and then rank them according to the octane, you end up with a very different scenario and value proposition:

If the number of available solution architects is truly your only constraint, you could run all the projects in green in parallel. In this case, only the last project cannot be completed. Therefore, the total NPV is $4.5bn, or a 67% improvement on scenario 1. Project 10, with its billion-dollar NPV, looked very attractive. Looking at project octane helps us see where true value lies.

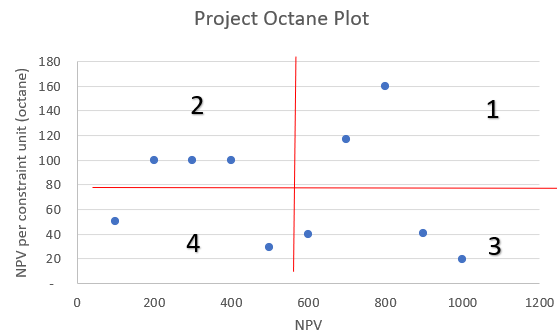
In the diagram above we can visualise this octane idea by plotting each project against two axes—the x axis representing the NPV and the y axis the project octane. The red lines indicate the average NPV and the average octane across all ten projects.

Quadrant 1: DYNAMO. These are the most desirable projects to do. They deliver the highest NPV and also have a high octane—that is, they are relatively low on consumption of the CCR and thus deliver a high bang for buck invested in them.

Quadrant 2: DREAMER. These projects have a relatively high octane (good bang for buck for the use of the CCR), but don’t generate enough NPV to move them into quadrant one. Some questions you could ask about these projects are: what can be done to increase the revenue (benefits) from these projects? If it’s a product or service offering, for example, would lowering the price lead to a significant and disproportionate increase in sales volume?

Quadrant 3: DELIVERER. These projects generate a relatively high NPV, but they are also proportionately more consumptive of the CCR than those projects in quadrant one. The question to ask of the projects in this quadrant is: what can be done to offload some or most of the work onto resources which are not as constrained as the solution architects? Perhaps the work can be looked at more closely and the constraint mantra applied: ‘let the constraint do only that which only the constraint can do’. What can be delegated to people who can support the CCR and thus liberate their capacity to do only that which only they can do?

Quadrant 4: DEADBEAT. As one wit put it, the projects in quadrant four are not only the dogs but are the fleas on the dogs! There would be two rounds of heavy-lifting to do to make these projects more attractive: increase the benefits and reduce the consumption of the CCR. It may be possible. But, once again, you have to ask yourself the opportunity cost question: where is your limited attention better spent? On the high-value projects or on wrestling the recalcitrant into submission?

I have found that the idea of project octane doesn’t have to be as quantitatively precise as expressed in these charts. It is best to set the intention to rise to a level of project maturity that makes the quantitative assessment second nature. But the truth is that good managers can usually get a pretty solid intuitive idea of octane without having to do the numbers. Just think about how ‘sticky’ or ‘viscous’ the project seems.

You would know that the numbers you get from a pure NPV calculation make you scratch your head and intuit that there is something not quite right—your critically constrained resources are spending too much time on a project when they could be cranking out a lot more, in a far more streamlined fashion, if allowed to work on those projects which consume less of their precious time. Using all of the tools that come from the ability to quantitatively articulate the load of your portfolio of work and the capacity available to deliver it can end up making you a lot more money, both now and in the future.

There is, of course, another option—the ‘uplift’ step of the 5-Step FOCUS. As one TOC guru put it, ‘when you’ve squeezed all the blood from a stone…get more stones’. Having the ability to quantitatively articulate the demand for resources, by type, in time and place, gives you the ability to pinpoint any further investment in capacity to provide the greatest leverage in value.

____________________________

What’s next?

The change from standard thinking to Theory of Constraints (TOC) is both profound and exhilarating. To make it both fun and memorable, we use a business simulation we call The Right Stuff Workshop.

We’d love to run it with you. To learn more:

• download the brochure (no email required)
• schedule a call____________________________

[Background photo: ‘Umbrellas’ by Ricardo Resende on Unsplash]

“Efficiency is doing things right;
effectiveness is doing the right things”
—Peter Drucker